Radiometry
MEASUREMENT GEOMETRIES, SOLID ANGLES
One of the key concepts to understanding the relationships between measurement geometries is that of the solid angle (ω). This can be defined as the angle that, seen from the center of the sphere, includes a given area on the surface of that sphere. The value of a solid angle is numerically equal to the size of the area on the surface of the sphere (A) divided by the square of the radius (r) of that sphere:
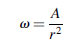 (5.4)
(5.4)A surface can be described as a continuum of infinitesimal points, each occupying an infinitesimal area dA,
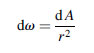 (5.5)
(5.5)where dω is the differential solid angle of the elemental cone containing a ray of light that is arriving at or leaving a infinitesimal surface dA. The symbol d stands for differential, the operator that reduces the applied variable to an infinitesimal quantity.
Most radiometric measurements do not require an accurate calculation of the spherical surface area. Flat area estimates can be substituted for spherical area when the solid angle is less than 0.03 steradians, resulting in an error of less than 1%. This roughly translates to a distance at least five times greater than the largest dimension of the detector. When the light source is the Sun, flat area estimates can be substituted for spherical area.
Light is radiant energy. When light is absorbed by a physical object, its energy is converted into some other form. Visible light causes an electric current to flow in a light detector when its radiant energy is transferred to the electrons as kinetic energy. Radiant energy (denoted as Q) is measured in joules (J).
SPECTRAL RADIANT ENERGY
A broadband source such as the Sun emits electromagnetic radiation throughout most of the electromagnetic spectrum. However, most of its radiant energy is concentrated within the PAR. A single-wavelength laser, on the other hand, is a monochromatic source; all of its radiant energy is emitted at one specific wavelength. From this, we can define spectral radiant energy, which is the amount of radiant energy per unit wavelength interval at wavelength λ. It is defined as:
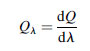 (5.6)
(5.6)Spectral radiant energy is measured in joules per nanometer (J nm-1).
Energy per unit time is power, which we measure in joules per second (J sec-1), or watts (W). Light “flows” through space and so radiant power is more commonly referred to as the flow rate of radiant energy with respect to time or radiant flux. It is defined as:
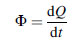 (5.7)
(5.7)where Q is radiant energy and t is time.
In terms of a light detector measuring PAR, the instantaneous magnitude of the electric current is directly proportional to the radiant flux. The total amount of current measured over a period of time is directly proportional to the radiant energy absorbed by the light detector during that time. For phycological purpose radiant flux is expressed also as micro moles of photons per second.
Spectral radiant flux at wavelength λ is radiant flux per unit wavelength interval. It is defined as:
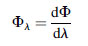 (5.8)
(5.8)and is measured in watts per nanometer (W nm-1).




