Randomization of treatments

For relatively simple experiments, you can adopt a completely randomized design; here, the position and treatment assigned to any subject is defined randomly. You can draw lots, use a random number generator on a calculator, or use the random number tables which can be found in most books of statistical tables.

A completely randomized layout has the advantage of simplicity but cannot show how confounding variables alter in space or time. This information can be obtained if you use a blocked design in which the degree of randomization is restricted. Here, the experimental space or time is divided into blocks, each of which accommodates the complete set of treatments. When analysed appropriately, the results for the blocks can be compared to test for differences in the confounding variables and these effects can be separated out from the effects of the treatments. The size and shape (or timing) of the block you choose is important: besides being able to accommodate the number of replicates desired, the suspected confounding variable should berelatively uniform within the block.

A Latin square is a method of placing treatments so that they appear in a balanced fashion within the experimental area. Treatments appear once in each column and row (see Fig. 10.2), so the effects of confounding variables can be 'cancelled out' in two directions at right angles to each other. This is effective if there is a smooth gradient in some confounding variable over the experimental area. It is less useful if the variable has a patchy distribution, where a randomized design might be better.

Latin square designs are useful in serial experiments where different treatments are given to the same subjects in a sequence (e.g. Fig. 10.3). A disadvantage of Latin squares is the fact that the number of columns and rows is equal to the number of replicates, so increases in the number of replicates can only be made by the use of further Latin squares.

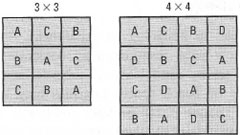Fig. 10.2 Examples of Latin square arrangements for three and four treatments. Letters indicate treatments; the number of possible arrangements increases greatly as the number of treatments increases.

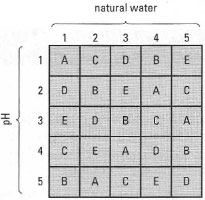Fig. 10.3 The experimenter wishes to investigate the effect of pHA-E on the extraction of phenols from five natural waters. Each water sample is extracted at a different pH and the influence of pH on the recovery of phenols noted.