The basis of a complexometric titration involving EDTA

The metal ion under investigation is bound to an indicator in solution (under strict pH control). This solution is then titrated against a standard solution of EDTA. This can be expressed in the form of an equation:
⇒Equation [23.1]

Metal-indicator + EDTA → metal-EDTA + indicator

For example, if the indicator being used was solochrome black, the metalindicator solution would be red while the colour of the free indicator would be blue (in the pH range 7-11). The reaction takes place if the EDTA displaces the indicator from the metal-indicator complex. Therefore the metal-EDT A complex must be more stable thermodynamically than the metal-indicator complex.

Stability of complexes
The thermodynamic stability of a species is an indication of the extent to which that species will be formed (under certain conditions and provided that it is allowed to reach equilibrium).

As an example consider the general case of a metal, M, in solution together with a monodentate ligand, L. It is possible to describe this system in terms of step-wise equilibria:

⇒Equation [23.2]	M + L = ML		K1 = [ML]/[M][L]
⇒Equation [23.3]	ML + L = ML2		K2 = [ML2]/[ML][L]

Or, in general terms:

⇒Equation [23.4]

ML(n − 1) + L = MLn

Kn = [MLn]/[ML(n − 1)][L]

where K₁, K₂,··· K_n are step-wise stability constants.

An alternative approach for expressing the equilibria might be as follows:

⇒Equation [23.5]	M + L = ML		β1 = [ML]/[M][L]
⇒Equation [23.6]	M + L2 = ML2		β2 = [ML2]/[M][L]2

Or, in general terms:

⇒Equation [23.7]

M + Ln = MLn

βn = [MLn]/[M][L]n

where β₁, β₂, ... , β_n are the overall stability constants and are related to the step-wise stability constants as follows:

⇒Equation [23.8]

βn = K1 × K2 × Kn

Factors influencing the stability of complexes
The stability of a complex is related to the ability of the metal ion to complex with a given ligand, and to the characteristics of the ligand.

End-points can be determined more easily when a single complex is formed rather than when the complex is formed in a step-wise fashion. This can be achieved by using the aminopolycarboxylic acid, EDTA (Fig. 23.2).

In equations, EDTA can be expressed as H4Y. The disodium salt Na₂H₂Y is frequently used as a source of the complex-forming ion, H₂Y²⁻. Thus the typical reaction of EDT A with a metal ion can be written in the following form:

⇒Equation [23.9]

M2+ + H2Y2− → MY2 + 2H+

The reaction of a metal ion with EDT A is always in the ratio I: I. The stability constants of selected metal-EDTA complexes are given in Table 23.1.

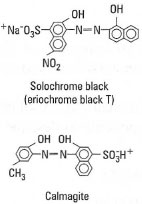Fig. 23.3 Examples of metal-ion indicators: solochrome black and calmagite.

The detection of the end-point in titrations involving EDTA is most commonly achieved using a metal-ion indicator, i.e. a compound that changes its colour when it complexes with a particular metal ion. The structures of selected metal-ion indicators are shown in Fig. 23.3 and the properties of a variety of metal-ion indicators are given in Table 23.2.

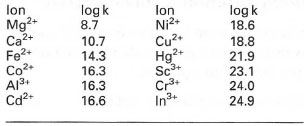Table 23.1 Stability constants of selected metal-EDTAcomplexes (expressed as log K)*

*Ionicstrength of solution was 0.1 at 20°C.